Chemistry Class 12 NCERT SOLUTION WITH Important Formula Ch 1' The Solid State '
The Solid State
Chapter–1
NCERT Textbook Questions
NCERT Intext Questions
Q. 1. Why are solids rigid?
Ans. In solids, the constituent particles (atoms or molecules or ions) are not free to move but can only oscillate
about their mean positions due to strong interatomic or intermolecular or interionic forces. This imparts
rigidity.
Q. 2. Why do solids have a definite volume?
Ans. The constituent particles in solids are bound to their mean positions by strong forces of attraction. The
interparticle distances remain unchanged at a given temperature and thus solids have a definite volume.
Q. 3. Classify the following as amorphous or crystalline solids:
Polyurethane, naphthalene, benzoic acid, teflon, potassium nitrate, cellophane, polyvinyl chloride,
fibre glass, copper.
Ans. Amorphous solids: Polyurethane, teflon, cellophane, polyvinyl chloride and fibre glass.
Crystalline solids: Naphthalene, benzoic acid, potassium nitrate and copper.
Q. 4. Why is glass considered a super cooled liquid?
Ans. Glass is an amorphous solid. Like liquids, it has a tendency to flow, though very slowly. This is evident
from the fact that the glass panes in the windows of old buildings are invariably found to be slightly thicker
at the bottom than at the top. This is because the glass flows down very slowly and makes the bottom
portion slightly thicker.
Q. 5. Refractive index of a solid is observed to have the same value along all directions. Comment on the nature of this solid. Would it show cleavage property?
Ans. Since the solid has the same value of refractive index along all directions, it is isotropic and hence,
amorphous. Being an amorphous solid, it would not show a clean cleavage when cut with a knife. Instead,
it would break into pieces with irregular surfaces.
Q. 6. Classify the following solids in different categories based on the nature of intermolecular forces operating in them:
Potassium sulphate, tin, benzene, urea, ammonia, water, zinc sulphide, graphite, rubidium, argon,
silicon carbide.
Ans. Ionic solids: Potassium sulphate, zinc sulphide.
Covalent solids: Graphite, silicon carbide.
Molecular solids: Benzene, urea, ammonia, water, argon.
Metallic solids: Rubidium, tin.
Q. 7. Solid A is a very hard electrical insulator in solid as well as in molten state and melts at extremely high temperature. What type of solid is it?
Ans. Covalent.
Q. 8. Ionic solids conduct electricity in molten state but not in solid state. Explain.
Ans. In the molten state, ionic solids ionise to give free ions and hence can conduct electricity. However, in the
solid state, since the ions are not free to move about but remain held together by strong electrostatic forces
of attraction, they behave as insulators.
Q. 9. What type of solids are electrical conductors, malleable and ductile?
Ans. Metallic solids.
Q. 10. Give the significance of a ‘lattice point’.
Ans. Each lattice point represents one constituent particle of the solid. This constituent particle may be an atom,
an ion, or a molecule.
Q. 11. Name the parameters that characterise a unit cell.
Ans. A unit cell is characterised by
(i) its dimensions along the three edges, a, b and c.
(ii) angles between the edges, which are a (between b and c), b (between a and c) and g (between a and b).
Thus, a unit cell is characterised by six parameters, a, b, c, a, b and g.
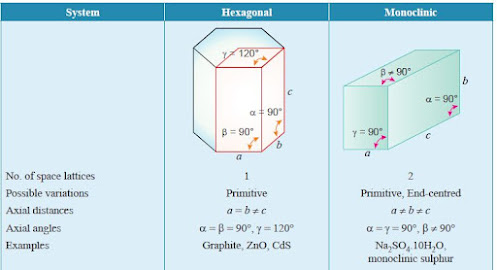
Q. 12. Distinguish between
(i) Hexagonal and monoclinic unit cells (ii) Face-centred and end-centred unit cells
Ans. (i) Hexagonal and monoclinic unit cells
Q. 13. Explain how much portion of an atom is located at (i) corner and (ii) body-centre of a cubic unit cell is part of its neighbouring unit cell.
Ans. (i) A point lying at the corner of a unit cell is shared equally by eight unit cells and therefore, only 1/8 of each such point belongs to the given unit cell.
(ii) A body-centred point belongs entirely to one unit cell since it is not shared by any other unit cell.
Q. 14. What is the two dimensional coordination number of a molecule in square close packed layer? [CBSE (F) 2013]
Ans. 4
Q. 15. A compound forms hexagonal close-packed structure. What is the total number of voids in 0.5 mol of it? How many of these are tetrahedral voids?
Ans. No. of atoms in the 0.5 mol close-packed structure = 0.5 × 6.022 × 1023 = 3.011 × 1023
No. of octahedral voids = 1 × No. of atoms in the close-packed structure = 3.011 × 1023
No. of tetrahedral voids = 2 × No. of atoms in the close-packed structure = 2 × 3.011 × 1023
= 6.022 × 1023
Total number of voids = 3.011 × 1023 + 6.022 × 1023 = 9.033 × 1023
Q. 16. A compound is formed by two elements, M and N. The element N forms ccp and atoms of M occupy 1/3rd of tetrahedral voids. What is the formula of the compound?
Ans. Suppose atoms of element N present in ccp = x
\ Number of tetrahedral voids = 2x
Since
1/3rd of the tetrahedral voids are occupied by atoms of element M,
number of atoms of element, M × x
x
3
1
2
3
2
` M = =
Ratio of M : N : :
x
x
3
2
= = 2 3
Hence, the formula of the compound = M2N3.
Q. 17. Which of the following lattices has the highest packing efficiency?
(i) simple cubic
(ii) body-centred cubic
(iii) hexagonal close-packed lattice.
Ans. Hexagonal close-packed lattice has the highest packing efficiency (74%).
Q. 18. An element with molar mass 2.7 × 10–2 kg mol–1 forms a cubic unit cell with edge length 405 pm. If its density is 2.7 × 103 kg m–3, what is the nature of the cubic unit cell?
Ans. Density, d = a N
z M
A
3 #
#
or z = M
d a NA
# 3 #
...(i)
Here, M = 2.7 × 10–2 kg mol–1
a = 405 pm = 405 × 10–12 m = 4.05 × 10–10 m
d = 2.7 × 103 kg m–3
NA = 6.022 × 1023 mol–1
Substituting these values in expression (i), we get
z =
.
( . ) (. )( . )
kg mol
kgm m mol
2 7 10
2 7 10 4 05 10 6022 10
–2 1
3 3 10 3 23 1
–
– – –
#
# # #
= 4
As there are 4 atoms of the element present per unit cell. Hence, the cubic unit cell must be face-centred.
Q. 19. What type of defect can arise when a solid is heated? Which physical property is affected by it and in
what way?
Ans. On heating a solid, vacancy defect is produced in the crystal. This is because on heating, some lattice sites
become vacant. As a result of this defect, the density of the substance decreases because some atoms or ions
leave the crystal completely.
Q. 20. What type of stoichiometric defect is shown by: (i) ZnS (ii) AgBr?
Ans. (i) ZnS shows Frenkel defect because its ions have a large difference in size.
(ii) AgBr shows both Frenkel and Schottky defects.
Q. 21. Explain how vacancies are introduced in an ionic solid when a cation of higher valence is added as an impurity in it.
Ans. When a cation of higher valence is added as an impurity in an ionic solid, some of the sites of the original cations are occupied by the cations of higher valency. Each cation of higher valency replaces two or more original cations and occupies the site of one original cation and the other site(s) remains vacant.
Q. 22. Ionic solids, which have anionic vacancies due to metal excess defect, develop colour. Explain with the help of a suitable example.
Ans. In ionic solids with anionic vacancies due to metal excess defect, when the metal atoms deposit on the surface, they diffuse into the crystal and after ionisation, the metal ion occupies cationic vacancy while electron occupies anionic vacancy. Such anionic sites occupied by an electron are known as F-centres.
These electrons get excited to higher energy levels by adsorption of suitable wavelengths from the visible white light and therefore appear coloured. When Na vapours are passed over NaCl crystals such defect is created and the crystals become yellow due to excess Na+ and presence of F-centres.
Q. 23. A group 14 element is to be converted into n-type semiconductor by doping it with a suitable impurity. To which group should this impurity belong?
Ans. n-type semiconductor means conduction due to presence of excess of electrons. Therefore, to convert group 14 element into n-type semiconductor, it should be doped with group 15 element e.g., As.
Q. 24. What type of substances would make better permanent magnets, ferromagnetic or ferrimagnetic? Justify your answer.
Ans. Ferromagnetic substances make better permanent magnets. This is because the metal ions of a ferromagnetic substance are grouped into small regions called ‘domains’. Each domain acts as a tiny magnet. These domains are randomly oriented. When a ferromagnetic substance is placed in a magnetic field, all the domains get oriented in the direction of the magnetic field and a strong magnetic field is produced. Such order of domains persists even when the external magnetic field is removed. Hence, the ferromagnetic substance becomes a permanent magnet. On the other hand, the net or resultant magnetic moment of ferrimagnetic substances is small.
NCERT Textbook Exercises
Q. 1. Define the term ‘amorphous’. Give a few examples of amorphous solids.
Ans. An amorphous solid consists of particles of irregular shape. The arrangement of constituent particles in such a solid has only short range order. In such an arrangement, a regular and periodically repeating pattern is observed over short distances only. Examples: Glass, rubber and plastics.
Q. 2. What makes a glass different from a solid such as quartz? Under what conditions could quartz be converted into glass?
Ans. Glass is an amorphous solid in which the constituent particles (SiO4 tetrahedra) have only a short range order and there is no long range order. In quartz, the constituent particles (SiO4 tetrahedra) have both short range as well as long range orders. On melting quartz and then cooling it rapidly, it is converted into glass.
Q. 3. Classify each of the following solids as ionic, metallic, molecular, network (covalent) or amorphous.
(i) Tetra phosphorus decoxide (P4O10) (ii) Ammonium phosphate (NH4)3PO4
(iii) SiC (iv) I2
(v) P4 (vi) Plastic
(vii) Graphite (viii) Brass
(ix) Rb (x) LiBr
(xi) Si
Ans. Ionic: (NH4)3PO4 and LiBr; Metallic: Brass, Rb; Molecular: P4O10, I2, P4;
Network (covalent): Graphite, SiC, Si; Amorphous: Plastic.
Q. 4. (i) What is meant by the term ‘coordination number’?
(ii) What is the coordination number of atoms: (a) in a cubic close packed structure? (b) in a body-centred cubic structure?
Ans. (i) Coordination number is defined as the number of nearest neighbours in a close-packed structure. In ionic crystals, coordination number of an ion in the crystal is the number of oppositely charged ions surrounding that particular ion.
(ii) (a) 12 (b) 8.
Q. 5. How can you determine the atomic mass of an unknown metal if know its density and the dimension of its unit cell? Explain your answer.
Ans. Refer to Basic Concepts Point 5. Atomicmass, M z
d×a ×NA
3
e = o
Q. 6. ‘Stability of a crystal is reflected in the magnitude of its melting point’. Comment. Collect melting points of solid water, ethyl alcohol, diethyl ether and methane from a data book. What can you say about the intermolecular forces between these molecules?
Ans. Higher the melting point, stronger are the forces holding the constituent particles together and hence greater
is the stability. In other words, stronger the lattice structure, higher will be lattice energy and more will
be the stability of crystal, hence higher the melting point. The intermolecular forces in water and ethyl
alcohol are mainly the hydrogen bonding. Higher melting point of water as compared to alcohol shows that
hydrogen bonding in ethyl alcohol molecules is not as strong as in water molecules. Diethyl ether is a polar
molecule and the intermolecular forces present in it is dipole–dipole attraction. Whereas methane is a nonpolar
molecule and the only forces present in it is the weak van der Waals’ forces.
Q. 7. How will you distinguish between the following pairs of terms: [CBSE (AI) 2014]
(i) Hexagonal close packing and cubic close packing?
(ii) Crystal lattice and unit cell?
(iii) Tetrahedral void and octahedral void?
Ans. (i) Refer to Basic Concepts Points 8(c) (i) and (ii).
(ii) The regular three dimensional arrangement of identical points in the space which represent how the constituent particles (atoms, ions, molecules) are arranged in a crystal is called a crystal lattice. A unit cell is the smallest portion of a crystal lattice, which when repeated over and again in different directions produces the complete crystal lattice.
(iii) A void surrounded by four spheres occupying the corners of tetrahedron is called a tetrahedral void. It
is much smaller than the size of spheres in the close packing. A void surrounded by six spheres along
the corners of an octahedral is called octahedral void. The size of the octahedral void is smaller than
that of the spheres in the close packing but larger than the tetrahedral void.
Q. 8. How many lattice points are there in one unit cell of each of the following lattices?
(a) face-centred cubic (b) face-centred tetragonal (c) body-centred.
Ans. (a) 14 (b) 14 (c) 9.
Q. 9. Explain:
(a) The basis of similarities and differences between metallic and ionic crystals.
(b) Ionic solids are hard and brittle.
Ans. (a) Similarities:
(i) Both ionic and metallic crystals have electrostatic forces of attraction. In ionic crystals, these are between the oppositely charged ions. In metals, these are among the valence electrons and the kernels.
(ii) In both cases, the bond is non-directional.
Differences:
(i) In ionic crystals, the ions are not free to move. Hence, they cannot conduct electricity in the solid state. They can do so only in the molten state or in aqueous solution. In metals, the valence electrons are not bound but are free to move. Hence, they can conduct electricity in the solid state.
(ii) Ionic bond is strong due to electrostatic forces of attraction. Metallic bond may be weak or strong depending upon the number of valence electrons and the size of the kernels.
(b) Ionic solids are hard because there are strong electrostatic forces of attraction among the oppositely charged ions. They are brittle because ionic bond is non-directional.
Q. 10. Calculate the efficiency of packing in case of a metal crystal for
(a) simple cubic (b) body-centred cubic (c) face-centred cubic
Ans. Do yourself
Q. 11. Silver crystallises in fcc lattice. If edge length of the cell is 4.07 × 10–8 cm and density is 10.5 g cm–3, calculate the atomic mass of silver.
Q. 12. A cubic solid is made up of two elements P and Q. Atoms of Q are at the corners of the cube and P at the body centre. What is the formula of the compound? What are the coordination numbers of P and Q?
Ans. Number of P atoms per unit cell = 1 (at the body centre) × 1 = 1
Number of Q atoms per unit cell = 8 (at the corners) ×1/ 8= 1
Hence the formula is PQ.
Coordination number of each of P and Q = 8.
Q.13. Niobium crystallises in body-centred cubic structure. If density is 8.55 g cm–3, calculate atomic radius of niobium using its atomic mass 93u.
Q. 15. Copper crystallises into a fcc lattice with edge length 3.61 × 10–8 cm. Show that the calculate density is in agreement with its measured value of 8.92 g cm–3.










Thanku for given such a good answers
ReplyDelete